Variance, covariance and correlation are the concepts of probability which can be described as the branch of mathematics that deals with the possible situations of random phenomena. In statistics and mathematics the measurement or calculation of mean, average, number in the data set, sum of the squares by number and values could be calculated by the different methods.
It explains the relationship of two random values at one point, their increasing and decreasing pattern to each other or independently. The complete discussion regarding these differences among variance, covariance and correlation will be elaborated in this article. Students will be able to learn the basic concept of variance, covariance, correlation and their difference among them.
Variance
In simple words, explaining the variance is “the fact of being different and inconsistent”. In mathematics it can be said as the average of square differences from the mean. It means it requires some of the calculations to calculate the difference between each point and the mean along with the square and average of the results.
For example if we have to calculate the mean of 20 numbers that ranges from 1 to 20 then its mean will be 10.5
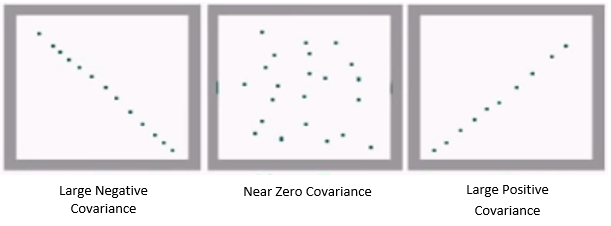
Covariance
As its name indicates, the covariance means “how two of the values or variables vary at one time”. In mathematics the covariance helps in measuring the relationship between the two random variables. Covariance evaluates the two variables at how much they change together. It can be measured in units and the final unit is calculated by multiplying both variable units. It also has two main types; the one is positive covariance in which the both variables tend to move in the same direction and the second one is negative covariance which means the both variables tend to move in the inverse direction.
Correlation
Correlation is referred to as the measure of the relationship between two variables and also that variables which move in the same direction. Correlation is also referred to as the measurement of strength of linear relationship between the two quantitative variables such as the weight of the variable and the height of the variable. It visually shows the both quantities direction and magnitude that vary together.
Difference between variance and covariance
Variance and covariance are the mathematical expressions that are frequently used in probability theory. The difference between the variance and covariance can be described as follows:
- Variance measures the data that would be scattered while the covariance measures the variation between the two random variables
- Variance is the spread of data that is set around its mean value while a covariance measures the directional relationship between the two variables.
- In the financial case, variance measures the assets volatility while the covariance describes the returning amount of two different investments over a period of time.
- Variance calculates the average and mean of the two values while the covariance increases or decreases the two quantities.
If you are still confused and to calculate covariance and variance easily within seconds and with steps use online sample covariance calculator and variance calculator probability for free .
Difference between covariance and correlation
Covariance and correlation are fundamentally the mathematical expression that evaluates the two random variables throughout the entire values. The difference between the covariance and correlation can be explained as follows:
- Covariance is the measure of variables that vary at one time while a correlation is the measure of two strong variables that relate to one another.
- Covariance can also be known as the measure of correlation while the correlation explains the scaled form of covariance
- Covariance specifies the direction of linear relationship between two variables while correlation measures the strength and direction of the linear relationship between two variables.
- Covariance concludes the unit from the both variables units after multiplying both of them while the correlation is unit free measure of the relationship between the variables.
- Covariance measures how much of the two dependent variables are in real quantity, e.g, in centimeters, kilograms and litres on the average that they co-vary while the correlation measures the proportion of two dependent variables that vary with respect to each other on average.
- Covariance is referred to as zero in terms of independent variables. For example the variables do not move together if only one variable moves and the next one is not while in correlation the independent movements have no contribution to the correlation and these independent variables have zero correlation.